If you are going to read all the information about number system on this page, then definitely read this article completely.
We have shared the post of Counting Chart on the previous page, so must read this post as well.
So let’s read and understand the definition of number system, all formulas of number system, types of number system, tricks to solve number system questions and questions related to number system with solutions.
What is Number System
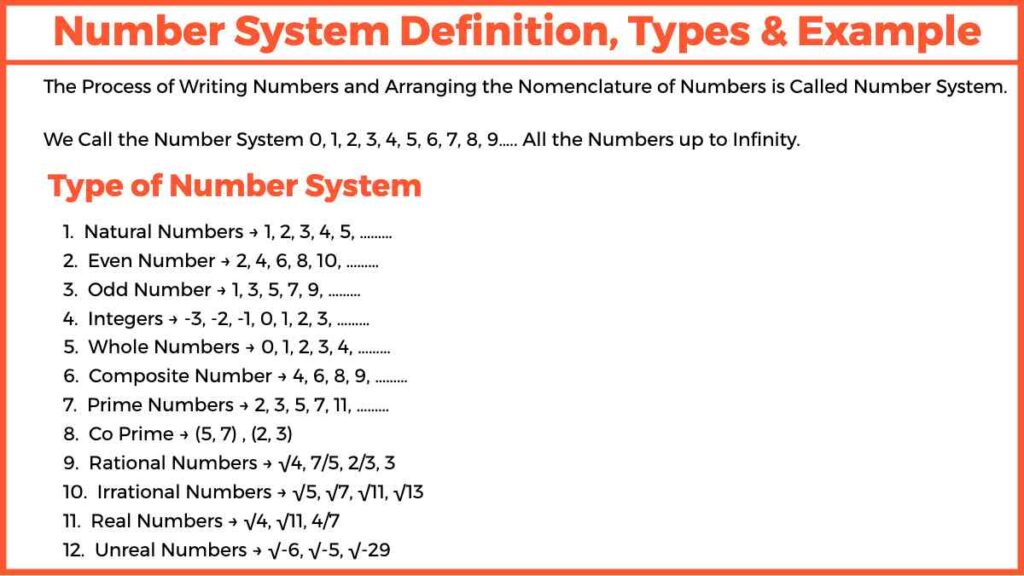
The process of writing numbers and arranging the nomenclature of numbers is called number system.
In number system, we study and understand numbers from 0 to infinity.
In the number system, we learn to use numbers by understanding the number of classes into which the numbers are divided.
We call the number system 0, 1, 2, 3, 4, 5, 6, 7, 8, 9….. all the numbers up to infinity.
Type of Number System
There are 12 types of numbers in the number system.
- Natural Numbers → 1, 2, 3, 4, 5, ………
- Even Number → 2, 4, 6, 8, 10, ………
- Odd Number → 1, 3, 5, 7, 9, ………
- Integers → -3, -2, -1, 0, 1, 2, 3, ………
- Whole Numbers → 0, 1, 2, 3, 4, ………
- Composite Number → 4, 6, 8, 9, ………
- Prime Numbers → 2, 3, 5, 7, 11, ………
- Co Prime → (5, 7) , (2, 3)
- Rational Numbers → √4, 7/5, 2/3, 3
- Irrational Numbers → √5, √7, √11, √13
- Real Numbers → √4, √11, 4/7
- Unreal Numbers → √-6, √-5, √-29
Below we read and understand the 12 types of numbers in detail.
1. Natural Number
Every number used in counting is called a Natural Number.
Example :- 1, 2, 3, 4, 5, 6, 7, 8, 9……………infinite
2. Even Number
Natural numbers which are exactly divisible by 2 are called Even Numbers.
Example :- 2, 4, 6, 8, 10, 12, 14, 16, 18, 20…….………infinite
3. Odd Number
Natural numbers which are not exactly divisible by 2 are called Odd Numbers.
Example :- 1, 3, 5, 7, 9, 11, ……………infinite
4. Integer Number
The numbers made up of positive, negative and zero are called Integer Numbers.
Example :- -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5……………..………infinite
There are three types of integer numbers.
- Positive Number
- Negative Number
- Neutral Integer
(a). Positive Numbers :- All positive numbers from one to infinity are positive integers.
(b). Negative Numbers :- All negative numbers from 1 to infinity are positive integers.
(c). Neutral Integer :- Such an integer on which no flow of positive and negative sign is read, then it is zero.
5. Whole Number
When zero is included in a natural number, it becomes a Whole Number.
Example :- 0, 1, 2, 3, 4, 5, 6, 7, 8, 9……….………infinite
6. Composite Number
A natural number which is divisible by at least one other number other than itself and 1 is called a Composite Number.
Example :- 4, 6, 8, 9, 10, 12, 14, 16, 18, 20……..………infinite
7. Prime Number
Natural numbers that are divisible only by themselves and 1 and not by any other number are called Prime Numbers.
Example :- 2, 3, 5, 11, 13, 17, ………
8. Co-prime Number
The set of at least 2 prime numbers whose HCF is 1 is called Co-Prime Number.
Example :- (5, 7), (2, 3)
9. Rational Number
All numbers that can be written in the form of p/q. They are called Rational Numbers.
(q denominator should not be zero)
Example :- 3/4, 7/12, 17/19, √125, √625
10. Irrational Number
Numbers that cannot be written in the form of p/q are usually written inside (√). And their perfect square root never comes out is called Irrational Number.
Example :- √13, √17, √123, √217, √257, √567
Note :- (Pi is an irrational number.)
11. Real Number
Real Numbers are obtained by writing rational and irrational numbers together.
Example :- 3/5, 7/11, 19/13, √121, √147, √973
12. Unreal Number
It is an imaginary number that does not have a real number. Unreal Numbers or imaginary numbers are represented by units.
Example :- √- 3, √-4, √-12, √-17, √-107 etc.
All Formulas of Number System
Formula Based on Numbers
- Sum of natural numbers = (first number + last number / 2) × n
- N = (last number – first number / class interval) + 1
- Sum of squares of first n natural numbers = n(n+1)(2n+1)/6
- Sum of cubes of first n natural numbers = [n(n+1)/2]²
Division Formulas
- Dividend = (Divisor × Quotient) + Remainder
- Dividend – Remainder = Divisor × Quotient
- Dividend – remainder / quotient = divisor
Formula Based on Number of Animals
- Number of four legs = (legs / 2) – head
- Number of two legs = Head – Number of four legs
Important Point Based on Unit Digit
- If there is 0 at the unit’s place, then the unit’s digit will be 0.
- If there is 1 at the unit’s place, then the unit’s digit will be 1.
- If there is 5 at the unit’s place, then the unit’s digit will be 5.
- If there is 6 at the unit’s place, then the unit’s digit will be 6.
Note :-
Trick 1. If the base number is 0, 1, 5, 6, unit digit respectively, the answer will also be 0, 1, 5, and 6 respectively.
Trick 2. If the unit digit of the numbers in the base is 2, 3, 4, 7, 8, 9, then divide the power by 4, and apply the remainder to the unit digit of the number written in the base.
Trick 3. 1, 2, and 3 will be obtained as remainders and 0 will be obtained when divided by perfection.
Number system based on place value and caste value
Place Value :- Two or more symbols are used in the number system based on place value. The number of symbols that are there is called the base of that number system. The values of these symbols range from zero to b-1 where b is the base.
In a given number –
Place value of unit digit = unit digit × 1
Place value of ten digit = ten digit × 10
Place value of hundreds digit = hundreds digit x 100
Place value of thousands digit = hundreds digit x 1000
Caste value :- Caste value of a digit in a given number is its own value, irrespective of its place.
Like :- in the number 567834
- The caste value of 3 is 3.
- The caste value of 6 is 6.
- The caste value of 8 is 8.
- The caste value of 4 is 4.
Number System Questions
Q.1 1 + 2 + 3 + 4 ……… 18 + 19 + 20 = ?
A. 210
B. 240
C. 280
D. 320
Solution : According to the question,
1 + 2 + 3 + 4 ……… 18 + 19 + 20 = ?
last number = 20
first number = 1
N = (last number – first number)/class interval + 1
N = (20 – 1)/1 + 1
N = 19 + 1
N = 20
Sum = (first number + last number / 2) × n
Sum = (1 + 20 )/2 × 20
Sum = (21 × 20)/2
sum = 21 × 10
sum = 210
Ans. 210
Q.2 1 + 2 + 3 + 4 ……… 78 + 79 + 80 = ?
A. 1,830
B. 3,240
C. 4,400
D. 3,860
Solution:- According to the question,
1 + 2 + 3 + 4 ……… 78 + 79 + 80 = ?
last number = 80
first number = 1
N = (last number – first number)/class interval + 1
N = (80 – 1)/1 + 1
N = 79 + 1
N = 80
Sum = (first number + last number / 2) × n
Sum = (1 + 80 )/2 × 80
Sum = (81 × 80)/2
sum = 81 × 40
sum = 3,240
Ans. 3,240
Q.3 What is the sum of all the numbers from 1 to 24?
A. 246
B. 262
C. 289
D. 300
Solution:- According to the question,
1 + 2 + 3 + 4 + …………….+ 20 + 21 + 22 + 23 + 24
first number = 1
last number = 24
N = (last number – first number)/class interval + 1
N = (24 – 1)/1 + 1
N = 23 + 1
N = 24
Sum of natural numbers = (first number + last number)/2 × n
Sum = (1 + 24)/2 × 24
Sum = (25 × 24)/2
Sum = 25 × 12
sum = 300
Ans. 300
Q.4 What is the sum of all the numbers from 1 to 72?
A. 2460
B. 2628
C. 2890
D. 3000
Solution:- According to the question,
1 + 2 + 3 + 4 + …………….+ 70 + 71 + 72
first number = 1
last number = 72
N = (last number – first number)/class interval + 1
N = (72 – 1)/1 + 1
N = 71 + 1
N = 72
Sum of natural numbers = (first number + last number)/2 × n
Sum = (1 + 72)/2 × 72
Sum = (73 × 72)/2
Sum = 73 × 36
sum = 2628
Ans. 2628
Q.5 What is the sum of all natural numbers from 21 to 75?
A. 2240
B. 2480
C. 2640
D. 2980
Solution:- According to the question,
21 + 22 + 23 + …………….+ 73 + 74 + 75
first number = 21
last number = 75
N = (last number – first number)/class interval + 1
N = (75 – 21)/1 + 1
N = 54 + 1
N = 55
Sum of natural numbers = (first number + last number)/2 × n
Sum = (21 + 75)/2 × 55
Sum = 96/2 × 55
Sum = 48 × 55
sum = 2640
Ans. 2640
Q.6 What is the sum of all natural numbers from 42 to 98?
A. 3,500
B. 3,587
C. 3,990
D. 4,160
Solution:- According to the question,
42 + 33 + 34 + …………….+ 94 + 95 + 98
first number = 42
last number = 98
N = (last number – first number)/class interval + 1
N = (98 – 42)/1 + 1
N = 56 + 1
N = 57
Sum of natural numbers = (first number + last number)/2 × n
Sum = (42 + 98)/2 × 57
Sum = 140/2 × 57
Sum = 70 × 57
sum = 3,990
Ans. 3,990
Q.7 What is the sum of all natural numbers from 50 to 100?
A. 2,270
B. 3,290
C. 1,780
D. 3,825
Solution:- According to the question,
50 + 51 + 52 + …………….+ 98 + 99 + 100
first number = 50
last number = 100
N = (last number – first number)/class interval + 1
N = (100 – 50)/1 + 1
N = 50 + 1
N = 51
Sum of natural numbers = (first number + last number)/2 × n
Sum = (50 + 100)/2 × 51
Sum = (150 × 51)/2
Sum = 75 × 51
sum = 3,825
Ans. 3,825
Q.8 What is the sum of all the natural numbers from 28 to 45?
A. 657
B. 720
C. 820
D. 900
Solution:- According to the question,
28 + 29 + 30 + …………….+ 43 + 44 + 45
first number = 28
last number = 45
N = (last number – first number)/class interval + 1
N = (45 – 28)/1 + 1
N = 17 + 1
N = 18
Sum of natural numbers = (first number + last number)/2 × n
Sum = (28 + 45)/2 × 18
Sum = (73 × 18)/2
Sum = 73 × 9
sum = 657
Ans. 657
Q.9 What is the sum of all natural numbers from 250 to 500?
A. 94,125
B. 72,034
C. 82,543
D. 99,234
Solution:- According to the question,
250 + 251 + 252 + …………….+ 498 + 499 + 500
first number = 250
last number = 500
N = (last number – first number)/class interval + 1
N = (500 – 250)/1 + 1
N = 250 + 1
N = 251
Sum of natural numbers = (first number + last number)/2 × n
Sum = (250 + 500)/2 × 251
Sum = (750 × 251)/2
Sum = 375 × 251
sum = 94,125
Ans. 94,125
Q.10 What is the sum of all natural numbers from 500 to 1000?
A. 9,84,125
B. 7,63,874
C. 3,75,750
D. 9,92,340
Solution:- According to the question,
500 + 501 + 502 + …………….+ 998 + 999 + 1000
first number = 500
last number = 1000
N = (last number – first number)/class interval + 1
N = (1000 – 500)/1 + 1
N = 500 + 1
N = 501
Sum of natural numbers = (first number + last number)/2 × n
Sum = (500 + 1000)/2 × 501
Sum = (1500 × 501)/2
Sum = 750 × 501
Sum = 3,75,750
Ans. 3,75,750
Q.11 What is the sum of all the even numbers from 2 to 50?
A. 720
B. 1404
C. 1234
D. 1664
Solution:- According to the question,
2 + 4 + 6 +…………..+ 46 + 48 + 50
N = (last number – first number)/2 + 1
N = (50 – 2)/2 + 1
N = 48/2 + 1
N = 24 + 1
N = 25
Sum = (first number + last number)/2 × n
Sum = (2 + 50)/2 × 25
Sum = 52/2 × 25
sum = 26 × 54
sum = 1404
Ans. 1404
Q.12 What is the sum of all the even numbers from 2 to 108?
A. 1,256
B. 2,208
C. 2,970
D. 3,250
Solution:- According to the question,
2 + 4 + 6 +…………..+ 104 + 106 + 108
N = (last number – first number)/2 + 1
N = (108 – 2)/2 + 1
N = 106/2 + 1
N = 53 + 1
N = 54
Sum = (first number + last number)/2 × n
Sum = (2 + 108)/2 × 54
Sum = 110/2 × 54
Sum = 55 × 54
sum = 2,970
Ans. 2,970
Q.13 What is the sum of all the even numbers from 28 to 180?
A. 6,230
B. 7,300
C. 8,008
D. 8,800
Solution:- According to the question,
28 + 30 + 32 + …………….+ 176 + 178 + 180
first number = 28
last number = 180
N = (last number – first number)/class interval + 1
N = (180 – 28)/2 + 1
N = 152/2 + 1
N = 76 + 1
N = 77
Sum of natural numbers = (first number + last number)/2 × n
Sum = (28 + 180)/2 × 77
Sum = (208 × 77)/2
sum = 104 × 77
sum = 8,008
Ans. 8,008
Q.14 What is the sum of all even numbers from 8 to 200?
A. 10,230
B. 10,300
C. 10,008
D. 10,088
Solution:- According to the question,
8 + 10 + 12 + …………….+ 196 + 198 + 200
first number = 8
last number = 200
N = (last number – first number)/class interval + 1
N = (200 – 8)/2 + 1
N = 192/2 + 1
N = 96 + 1
N = 97
Sum of natural numbers = (first number + last number)/2 × n
Sum = (8 + 200)/2 × 97
Sum = (208 × 97)/2
sum = 104 × 97
sum = 10,088
Ans. 10,088
Q.15 What is the sum of all the even numbers from 112 to 240?
A. 11,330
B. 12,300
C. 11,440
D. 12,880
Solution:- According to the question,
112 + 113 + 114 + …………….+ 238 + 239 + 240
first number = 112
last number = 240
N = (last number – first number)/class interval + 1
N = (240 – 112)/2 + 1
N = 128/2 + 1
N = 64 + 1
N = 65
Sum of natural numbers = (first number + last number)/2 × n
Sum = (112 + 240)/2 × 65
Sum = (352 × 65)/2
sum = 176 × 65
sum = 11,440
Ans. 11,440
Q.16 Find the sum of all the odd numbers from 1 to 69?
A. 1225
B. 1480
C. 1660
D. 1870
Solution:- According to the question,
1 + 3 + …………….+ 67 + 69
first number = 1
last number = 69
N = (last number – first number)/class interval + 1
N = (69 – 1)/2 + 1
N = 68/2 + 1
N = 34 + 1
N = 35
Sum of natural numbers = (first number + last number)/2 × n
Sum = (1 + 69)/2 × 35
Sum = 70/2 × 35
sum = 35 × 35
sum = 1225
Ans. 1225
Q.17 What is the sum of all odd numbers from 23 to 71?
A. 1176
B. 1175
C. 1178
D. 1179
Solution:- According to the question,
23 + 25 + 27 + 29 + …………….+ 67 + 68 + 71
first number = 23
last number = 71
N = (last number – first number)/class interval + 1
N = (71 – 23)/2 + 1
N = 48/2 + 1
N = 24 + 1
N = 25
Sum of natural numbers = (first number + last number)/2 × n
Sum = (23 + 71)/2 × 25
Sum = 94/2 × 25
Sum = 47 × 25
sum = 1175
Ans. 1175
Q.18 What is the sum of squares of all natural numbers from 1 to 20?
A. 1,738
B. 2,870
C. 2,872
D. 2,827
Solution:- According to the question,
n = 20
Sum = n (n + 1) (2n + 1) / 6
Sum = 20 (20 + 1)(2 × 20 + 1) / 6
Sum = 20 × 21 (40 + 1) / 6
Sum = (20 × 21 × 41) / 6
sum = 10 × 7 × 41
sum = 2,870
Ans. 2,870
Q.19 What is the sum of squares of all natural numbers from 1 to 80?
A. 1,71,880
B. 1,71,880
C. 1,73,880
D. 1,74,880
Solution:- According to the question,
n = 80
Sum = n (n + 1) (2n + 1) / 6
Sum = 80 (80 + 1)(2 × 80 + 1) / 6
Sum = 80 × 81 (160 + 1) / 6
Sum = 80 × 81 × 161 / 6
Sum = 1,73,880
Ans. 1,73,880
Q.20 What is the sum of squares of all natural numbers from 1 to 32?
A. 10,580
B. 11,440
C. 12,640
D. 14,720
Solution: According to question,
n = 32
Sum = n (n + 1) (2n + 1 ) / 6
Sum = 32 (32 + 1)(2 × 32 + 1) / 6
Sum = 32 × 33 (64 + 1) / 6
Sum = (32 × 33 × 65) / 6
sum = 16 × 11 × 65
sum = 11,440
Ans. 11,440
Q.21 What is the sum of squares of all natural numbers from 1 to 10?
A. 385
B. 450
C. 520
D. 620
Solution: According to question,
n = 10
Sum = n (n + 1) (2n + 1) / 6
Sum = 10 (10 + 1) (2 × 10 + 1) / 6
Sum = 10 × 11 (20 + 1) / 6
Sum = (10 × 11 × 21) / 6
Sum = (11 × 7 × 5) / 6
sum = 385
Ans. 385
Q.22 What is the sum of cubes of all natural numbers from 1 to 100?
A. 25,502,500
B. 25,578,900
C. 25,890,800
D. 25,690,025
Solution:- According to the question,
[n (n + 1) / 2]²
n = 100
[100 (100 + 1) / 2]²
[(100 × 101) / 2]²
(50 × 101)²
(5,050)²
25,502,500
Ans. 25,502,500
Q23. What is the unit digit of 63 × 92 × 43 × 109 × 69?
A. 1
B. 2
C. 4
D. 8
Solution:- According to the question,
unit digit
= 3 × 2 × 3 × 9 × 9
= 18 × 81
= 8 × 1
The unit digit will be 8.
Ans. 8
Q.24 (32589)^1616
A. 1
B. 3
C. 5
D. 7
Solution:- According to the question,
1616/4
On dividing the number by 4, the remainder remains 0, so here we will raise the unit’s digit to the power of 4.
(9)^4
9×9×9×9
81 × 81
1×1
Hence the unit digit will be 1.
Ans. 1
Q.25 (879345)^2836
A.1
B.3
C.5
D.7
Solution:- According to the question,
2836/4
On dividing the number by 4, the remainder remains 0, so here we will raise the unit’s digit to the power of 4.
(5)^4
5×5×5×5
25×25
5 × 5
25
Hence the unit digit will be 5.
Ans. 5
Q.26 What is the place value of 6, 2 in the number 4562?
Solution:- In the number 4562,
Place value of 2 ⇒ 2 × 1 = 2
Place value of 6 ⇒ 6 × 10 = 60
Q.27 What is the place value of the digits 4, 6, 3 in the number 45623?
Solution:- In the number 45623,
Place value of 3 ⇒ 3 × 1 = 6
Place value of 6 ⇒ 6 x 100 = 600
Place value of 4 ⇒ 4 x 10000 = 40000
Q.28 Write the place value of the following digits in the number 12645890?
Solution:- In the number 12645890,
Place value of 0 ⇒ 0 × 1 = 0
Place value of 9 ⇒ 9 × 10 = 90
Place value of 8 ⇒ 8 x 100 = 800
Place value of 5 ⇒ 5 × 1000 = 5000
Place value of 4 ⇒ 4 x 10000 = 40000
Place value of 6 ⇒ 6 x 100000 = 600000
Place value of 2 ⇒ 2 × 1000000 = 2000000
Place value of 1 ⇒ 1 × 10000000 = 10000000
Q.29 Write the place value of the following digits in the number 481235?
Solution:- In the number 481235,
Place value of 5 ⇒ 5 × 1 = 5
Place value of 3 ⇒ 3 × 10 = 30
Place value of 2 ⇒ 2 x 100 = 200
Place value of 1 ⇒ 1 × 1000 = 1000
Place value of 8 ⇒ 8 x 10000 = 80000
Place value of 4 ⇒ 4 x 100000 = 400000
Q.30 Write the place value of the following digits in the number 32567809?
Solution:- In the number 32567809,
Place value of 9 ⇒ 9 × 1 = 9
Place value of 0 ⇒ 0 × 10 = 00
Place value of 8 ⇒ 8 x 100 = 800
Place value of 7 ⇒ 7 × 1000 = 7000
Place value of 4 ⇒ 4 x 10000 = 40000
Place value of 6 ⇒ 6 x 100000 = 600000
Place value of 2 ⇒ 2 × 1000000 = 2000000
Place value of 1 ⇒ 1 × 10000000 = 10000000
Q.31 Write the caste value of the following digits in the number 78345?
Solution:- In the number 78345,
Caste value of 7 = 7
Caste value of 8 = 8
Caste value of 3 = 3
Caste value of 4 = 4
Caste value of 5 = 5
Q.32 Write the caste value of the following digits in the number 536487?
Solution:- In the number 536487,
Caste value of 5 = 5
Caste value of 3 = 3
Caste value of 6 = 6
Caste value of 4 = 4
Caste value of 8 = 8
Caste value of 7 = 7
Q.33 The average of three consecutive even numbers is 24, then find the smallest even number?
A.20
B.10
C.40
D.22
Solution:- According to the question,
Three consecutive even numbers – 22, 24, 26
Smallest even number = 22
Ans. 22
Q.34 The sum of five consecutive even numbers is 80. So tell which is the largest even number?
A.10
B.20
C.15
D.30
Solution:- According to the question,
Sum of five consecutive even numbers 80
= 80/5
= 16
Five consecutive even numbers – 12, 14, 16, 18, 20
Largest even number = 20
Ans. 20
Q.35 The sum of five consecutive even numbers is 250. Then what will be the square of the sum of the smallest and the largest number?
A.1000
B.10000
C.100
D.20000
Solution:- According to the question,
The sum of five consecutive even numbers is 250.
= 250/5
= 50
Five consecutive even numbers – 46, 48, 50, 52, 54
square of the sum of the smallest and the largest number
46 + 54
(100)²
10000
Ans. 10000
Q.36 The sum of five consecutive odd numbers is 145. Then find the square of the smallest odd number?
A.625
B.620
C.630
D.650
Solution:- According to the question,
The sum of five consecutive odd numbers is 145.
= 145/5
= 29
Five consecutive odd numbers – 25, 27, 29, 31, 33
Square of the smallest odd number = (25)²
625
Ans. 625
Q.37 The sum of five consecutive odd numbers is 55. So what is the product of the multiplication of the largest and the smallest number?
A.100
B.105
C.110
D.120
Solution:- According to the question,
The sum of five consecutive odd numbers is 55.
= 55/5
= 11
Five consecutive odd numbers – 7, 9, 11, 13, 15
Product of the largest and smallest number = 7 × 15
105
Ans. 105
Q.38 The sum of four consecutive even numbers is 36. What is the third largest number?
A.5
B.10
C.15
D.20
Solution:- According to the question,
The sum of four consecutive even numbers is 36.
= 36/4
= 9
Four consecutive even numbers – 6, 8, 9, 10, 12
Third largest number = 10
Ans. 10
Q.39 The sum of six consecutive even numbers is 102. What is the square of the smallest number?
A.140
B.144
C.150
D.156
Solution:- According to the question,
The sum of six consecutive even numbers is 102.
= 102/6
= 17
Six consecutive even numbers – 12, 14, 16, 17, 18, 20, 22
Square of the smallest number = (12)²
= 144
Ans. 144
Q.40 The sum of four consecutive odd numbers is 72. What is the difference between the largest and the smallest number?
A.4
B.6
C.9
D.12
Solution:- According to the question,
The sum of four consecutive odd numbers is 72.
= 72/4
= 18
Four consecutive odd numbers – 15, 17, 18, 19, 21
Difference of largest and smallest number = 21 – 15
6
Ans. 6
Q.41 The sum of six consecutive odd numbers is 180. Then what will be the square of the fourth largest number?
A.900
B.921
C.961
D.990
Solution:- According to the question,
The sum of six consecutive odd numbers is 180.
= 180/6
= 30
6 consecutive odd numbers – 25, 27, 29, 30, 31, 33, 35
Square of 4th largest number = (31)²
961
Ans. 961
Q.42 A cart was carrying its sheep if the total number of feet on the ground is 230. And the total number of heads is 60, then what is the total number of sheep he has?
A.50
B.55
C.60
D.65
Solution:- According to the question,
Number of four legs = (legs/2) – head
= (230/2) – 60
= 115 – 60
= 55
Ans. 55
Q.43 Some parrots are sitting on a tree. And some goats are specially climbing under the tree. So the number of heads is 35. The total number of legs is 90. What is the total number of parrots and goats?
A.20
B.25
C.30
D.35
Solution:- According to the question,
Number of four legs = legs/2 – head
= 90/2 – 35
= 45 – 35
= 10
Number of two legs = Head – Number of four legs
= 35 – 10
= 25
Ans. 25
Q.44 There are some rats and pigeons in a zoo. If the total number of heads is 90 and the total number of legs is 224. So tell what is the total number of pigeons?
A.68
B.70
C.72
D.76
Solution:- According to the question,
Number of four legs = legs/2 – head
= 224/2 – 90
= 112 – 90
= 22
Number of two legs = Head – Number of four legs
= 90 – 22
= 68
Ans. 68
Q.45 A person has some hens and cows. If the total number of their legs is 140 and the total number of their heads is 48, then what is the number of chickens?
A.22
B.26
C.28
D.30
Solution:- According to the question,
Number of four legs = legs/2 – head
= 140/2 – 48
= 70 – 48
= 22
Number of two legs = Head – Number of four legs
= 48 – 22
= 26
Ans. 26
Q.46 In a division problem, the quotient is 403. And if the denominator is 100. And the remainder is 58, then what is the dividend?
Solution:- According to the question,
Dividend = ?
Quotient = 403
remainder = 58
divisor = 100
Dividend = (Divisor × Quotient) + Remainder
Dividend = (100 × 403) + 58
Dividend = 40300 + 58
Dividend = 40358
Ans. 40358
FAQ
Ans. a system to represent or express numbers.
Ans. A system for representing (that is expressing or writing) numbers of a certain type.
Example : There are several systems for representing the counting numbers. These include: The usual “base ten” or “decimal” system: 1, 2, 3, … , 10, 11, 12, …
Ans. An Indian mathematician Aryabhatta is the father of the number system
Ans. Zero’s real name is Hector Zeroni, but he has been called Zero for most of his life. He has been homeless for most of his life, and his mother abandoned (or lost) him when he was still small.
Ans. Aryabhata, a great astronomer of the classic age of India was the one who invented the digit “0” (zero) for which he became immortal but later on is given to Brahmagupta who lived around a century later 22, another ancient Indian mathematician.
Hope you have liked the information about number system. If you liked the number system, then do share it with your friends.
If you want to ask any question related to number system, then definitely ask in the comment section. Each question related to number system will be answered in the comments.