On this page, you are going to read all the information about important chapters of Mathematics, Ratio and Proportion like: definition, formula, tricks and examples of ratio and proportion etc.
If you are new to the blog, then we would like to tell you that we have shared information about all the Chapters of Mathematics and Formulas of Mathematics for you, by reading which you can easily prepare for the mathematics subject.
Let us first read and understand the information about Ratio and Proportion.
Definition of Ratio
“The comparative study of two similar quantities is called ratio, if a and b are two non-zero Numbers, then the ratio of a and b is denoted by a : b and a is read as ratio b.”
When two like quantities are compared by the operation of Division, the quotient obtained is called a Ratio.
In other words we can say that “Ratio is a number that forms the relationship between two homogeneous quantities which shows how many times more or less one quantity is than the other.”
The Symbol : is used to Display the Ratio.
If, a and b are two homogeneous quantities. So, the ratio of a and b is written as a/b or a : b and the ratio of a to b is read.
Both the quantities in the ratio are called Post, the first quantity is called former post or First Post and the second quantity is called Uttar Post or Second Post.
Features of Ratio
- Ratio is a numerical relationship, hence it has no units.
- The ratio of two zodiac signs is a fraction, the numerators of which are the first zodiac sign and every second zodiac sign.
- When both terms of a ratio are multiplied or divided by the same non-zero number, the value of the ratio remains unchanged.
- By adding or subtracting the same non-zero number to both the terms of a ratio, the value of the ratio changes.
- Ratio is always taken for homogeneous quantities.
Types of Ratio
Mainly there are 6 types of ratios which are given below.
1. Simple Ratio
If both the terms of a ratio are co-prime to each other, then such ratio is called a simple ratio.
Example :- 2 : 3
2. Compound Ratio
A new ratio formed by multiplying the products of the first terms and the last terms of two or more ratios is called mixed ratio.
Example :- The mixed ratio of two ratios (a : b) and (c : d) will be (ac : bd). Similarly, the mixed ratio of 2 : 3, 4 : 5 and 6 : 7 will be 2 × 4 × 6 : 3 × 5 × 7 i.e. 48 : 105 or 16 : 35.
3. Inverse Ratio or Reciprocal
The new ratio obtained by reversing the terms of a ratio is called inverse ratio.
Example :- The converse ratio of 2 : 3 will be 3 : 2.
4. Duplicate Ratio
If a new ratio is created by mixing any ratio with itself, then it is called square ratio.
Example :- Square ratio of 2 : 3 is 2² : 3³
That means 2 × 2 will be : 3 × 3 or 4 : 9.
Similarly, 2² : 3³ is called triple ratio, √2 : √3 is called half ratio, 3√2 : 3√3 is called third ratio.
5. Fourth Ratio
If four non-zero quantities a, b, c and d are in proportion, then d is called quadratic proportional to a, b, c.
6. Financed Ratio
Three non-zero numbers a, b and c will be in direct proportion.
If a/b = b/c
If a, b and c are in direct proportion, then b² = ac
Here, b is called mean proportional and c is called third proportional.
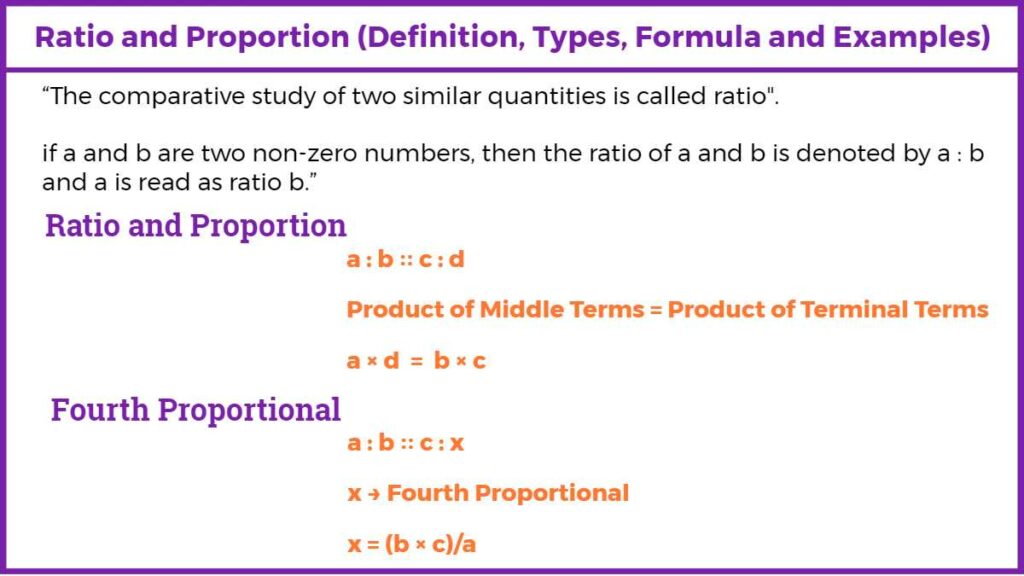
Definition of Proportion
Out of the four zodiac signs, when the ratio of the first zodiac sign and the second zodiac sign is equal to the ratio of the third and fourth zodiac signs, then it is called proportionality.
Example :- a : b : : c : d
If there are four non-zero numbers a, b, c and d such that a : b = c : d, then a, b, c and d are in proportion.
If a : b :: c : d, then ad : b c. In this, a and d are called outer terms and b and c are called middle terms.
Types of Proportion
1. Constant Proportion
If three numbers a, b and c are in constant proportion, then we can say that a, b and c are in proportion.
So, a/b = b/c
b² = ac
b = √ac
Hence we can say that a is the first proportion, c is the third proportion and b is the middle proportion.
2. Direct Proportion
If X is directly proportional to Y, then increase or decrease in one will have a direct impact on the other.
If X increases then Y will also increase and if X decreases then Y will also decrease.
3. Inverse Proportion
If Y decreases when X increases and Y increases when X decreases, then this proportion is called inverse proportion.
If four quantities are in proportion, then the product of the quantities at the edge is equal to the product of the quantities in the middle.
Let four quantities a, b, c, d be in proportion, then a/b = c/d
then ad = b c
If three quantities a, b and c are in constant proportion, then a : b = b : c
then ac = b²
b is called middle proportion.
If three zodiac signs are in proportion, then the ratio of the first and third zodiac signs is the same as the ratio of the first and second zodiac signs.
If a : b : : b : c then a : c = a² : b²
Answers Related to Ratio and Proportion
Q.1 What will be the first proportional of 4 and 9?
A. 1.2
B. 1.4
C. 1.7
D. 2.0
Solution:- According to the question,
a = b² / c
a = (4)² / 9
a = 16/9
a = 1.7
Ans. 1.7
Q.2 What will be the middle proportional of 8 and 2?
A. 2
B. 4
C. 6
D. 8
Solution:- According to the question,
b = √ac
b = √8 × 2
b = √16
Ans. 4
Q.3 What will be the third proportional of 4 and 9?
A. 10.40
B. 12.50
C. 20.25
D. 25.30
Solution:- According to the question,
c = b² / a
c = 9² / 4
c = (9 × 9) / 4
c = 81/4
Ans. 20.25
Q.4 What will be the first proportional of 4 and 8?
A. 2
B. 4
C. 6
D. 8
Solution:- According to the question,
a = b²/c
a = (4)²/8
a = 16/8
a = 2
Ans. 2
Q.5 What will be the middle proportion of 0.4 and 0.9?
A. 0.2
B. 0.4
C. 0.6
D. 0.8
Solution:- According to the question,
b = √ac
b = √0.4 × 0.9
b = √0.36
b = 0.6
Ans. 0.6
Q.6 9 : 15 : : 45 : ?
A. 3
B. 9
C. 27
D. 81
Solution:- According to the question,
9 : 15 : : 45 : ?
9/15 = 45/x
9 × x = 45 × 15
x = (45 × 15)/9
x = 15 × 5
x = 75
Ans. 75
Q.7 5 : ? , :125
A. 20
B. 25
C. 30
D. 35
Solution:- According to the question,
5 : ? , :125
?² = 125 × 5
?² = 625
? = 25
Ans. 25
Q.8 5 : 8 :: 150 : x
A. 200
B. 220
C. 240
D. 260
Solution:- According to the question,
5 × x = 150 × 8
x = (150 × 8)/5
x = 30 × 8
x = 240
Ans. 240
Q.9 3 : 5 :: 21 : ?
A. 30
B. 35
C. 40
D. 50
Solution:- According to the question,
3 : 5 : : 21 : ?
3 × ? = 21 × 5
? = (21 × 5)/3
? = 7 × 5
? = 35
Ans. 35
Q.10 Tell the first proportional of 4, 48 and 16?
A. 10
B. 12
C. 14
D. 16
Solution:- According to the question,
x : 4 : 48 : 16
x × 16 = 4 × 48
x = (4 × 48) / 16
x = 12
Ans. 12
FAQ
Ans. The relationship in quantity or degree between two things:
Ans. A ratio is a comparison of two quantities. A proportion is an equality of two ratios.
Ans. when both sides are whole numbers and there is no whole number that both sides can be divided by.
Ans. Ratio is used for comparing two quantities of the same kind.
Ans. the square root (such as x) of the product of two numbers (such as a and b) when expressed as the means of Aproportion (such as a/x = x/b)
Hope you will like the information about ratio and proportion and after reading it you will be able to learn to solve the problems of ratio and proportion.
Comment for any Question Related to Ratio and Proportion.
If you liked this Post, then share it with your friends on Facebook and LinkedIn etc.
Very Informative article! Keep posting!
THANKS