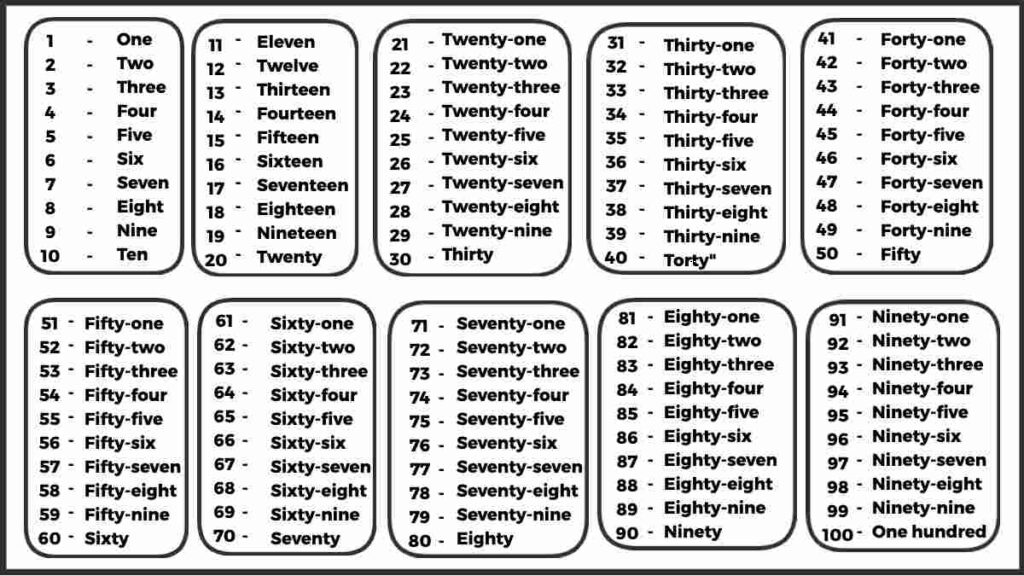
In this article, you will read the Definition, Formula, sum and solution of important questions of Natural Numbers.
On the previous page, we have shared the post of Number System, an important chapter of mathematics, so definitely read the square root.
Let us read and understand the definition, formulas, tricks, and examples of natural numbers on this page.
What are Natural Number
All Numbers used in Counting are called Natural Numbers.
Ex : 1, 2, 3, 4, 5, 6, 7, 8, 9………
Natural Numbers are Denoted by N.
The Numbers which are used to count an object are called natural numbers.
As :-
- There are 5 books kept in the bag.
- There are 4 pens on the table.
- There are 30 students studying in a Mathematics class.
- Natural numbers are also used for ordering.
As :-
- Ram stood first in class 10th.
- Sita secured 4th rank in Law.
- Shyamu sits sixth in the class.
Formulas for Natural Numbers
- Average of first n natural odd numbers = n
- Average of first n natural even numbers = n + 1
- Sum of natural numbers = (first number + last number / 2) × n
- Average of first n natural numbers = ( n + 1 ) / 2
- Average of n consecutive odd natural numbers = (n + 1) / 2
- Sum of consecutive odd natural numbers up to n = (n/2 + 1)
- N = (last number – first number / class interval) + 1
- Sum of squares of first n natural numbers = n( n + 1 )( 2n + 1 ) / 6
- Sum of cubes of first n natural numbers = [ n (n + 1) / 2 ]²
Important Point :
- The smallest natural number is 1.
- The largest natural number is infinity.
- In the set, 0 is considered a natural number.
- Natural number are a part of number system.
- Natural number are represented by N.
- Where are natural number known as Hindi Arabic numbers on the basis of ancient history.
- Natural numbers keep on increasing by 1 unit continuously.
- Natural numbers = 0 < N ≤ 1.
- The set is denoted by N0 = {1, 2, 3, 4, 5, 6, 7, 8, 9, ……).
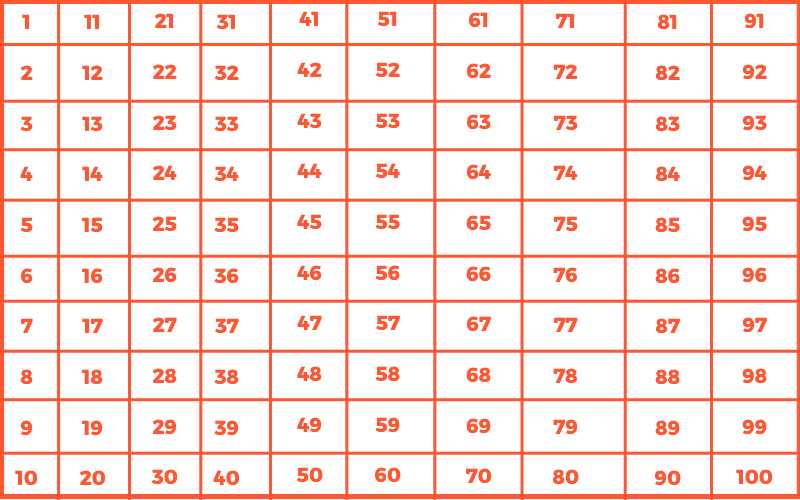
Natural Number From 1 to 100
Sum of First 10 Natural Number
First 10 natural number = 1, 2, 3, 4, 5, 6, 7, 8, 9, 10
Sum of first 10 natural number = 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10
Sum of first 10 natural number = 55
Hence the sum of first 10 natural number is 55.
Definition of Square : When a number is multiplied by the same number, the product obtained is called the square of the number.
What is the average of the squares of the first 10 natural number?
Square from 1 to 10
| 1² | 1 |
| 2² | 4 |
| 3² | 9 |
| 4² | 16 |
| 5² | 25 |
| 6² | 36 |
| 7² | 49 |
| 8² | 64 |
| 9² | 81 |
| 10² | 100 |
Sum of squares of first 10 natural number = 1 + 4 + 9 + 16 + 25 + 36 + 49 + 64 + 81 + 100
Sum of squares of first 10 natural number = 385
Questions Based on Natural Number
Q.1 1 + 2 + 3 + 4 ……………… 18 + 19 + 20 = ?
A. 120
B. 210
C. 240
D. 320
Solution : According to the question,
1 + 2 + 3 + 4 ……………… 18 + 19 + 20 = ?
last number = 20
first number = 1
N = (last number – first number)/class interval + 1
N = ( 20 – 1 ) / 1 + 1
N = 19 + 1
N = 20
Sum = (first number + last number / 2) × n
Sum = (1 + 20 ) / 2 ×20
Sum = 21 × 20 / 2
sum = 21 × 10
sum = 210
Ans. 210
Q.2 1+2+3+4 ……………… 78+79+80 = ?
A. 1,220
B. 1,880
C. 3,240
D. 4,860
Solution : According to the question,
1+2+3+4 ……………… 78+79+80 = ?
last number = 80
first number = 1
N = (last number – first number)/class interval + 1
N = ( 80 – 1 ) / 1 + 1
N = 79 + 1
N = 80
Sum = (first number + last number / 2) × n
Sum = (1 + 80 ) / 2 × 80
Sum = 81 × 80 / 2
sum = 81 × 40
sum = 3,240
Ans. 3,240
Q.3 What is the sum of all the odd numbers from 1 to 49?
A. 360
B. 625
C. 720
D. 800
Solution : According to the question,
1 + 3 + …………….………+ 48 + 49
first number = 1
last number = 49
N = (last number – first number)/class interval + 1
N = (49 – 1)/2 + 1
N = 48/2 + 1
N = 24 + 1
N = 25
Sum of natural number = (first number + last number)/2 × n
Sum = (1 + 49)/2 × 25
Sum = 50/2 × 25
Sum = 25 × 25
sum = 625
Ans. 625
Q.4 What is the sum of squares of all natural number from 1 to 20?
A. 1,385
B. 4,350
C. 2,260
D. 2,870
Solution : According to the question,
n = 20
Sum = n (n + 1) (2n + 1) / 6
Sum = 20 (20 + 1) (2 × 20 + 1) / 6
Sum = 20 × 21 (40 + 1) / 6
Sum = (20 × 21 × 41) / 6
Sum = 17,220 / 6
sum = 2,870
Ans. 2,870
Q.5 What is the sum of squares of all natural number from 1 to 80?
A. 1,37,895
B. 1,24,350
C. 2,26,670
D. 1,73,880
Solution : According to the question,
n = 80
Sum = n (n + 1) (2n + 1) / 6
Sum = 80 (80 + 1) (2 × 80 + 1) / 6
Sum = 80 × 81 (160 + 1) / 6
Sum = (80 × 81 × 161) / 6
Sum = 1,043,280 / 6
Sum = 1,73,880
Ans. 1,73,880
Q.6 What is the sum of cubes of all natural numbers from 1 to 40?
A. 468,900
B. 672,400
C. 678,908
D. 566,902
Solution : According to the question,
[n (n + 1) / 2]²
n = 40
[40 (40 + 1) / 2]²
(40 × 41 / 2)²
(1,640 / 2)²
(820)²
Ans. 672,400
Q.7 The number obtained by subtracting four times the number from thrice the square of a natural number is 50 more than that number. is that number?
A. 4
B. 5
C. 6
D. 10
Solution : According to the question,
Let x be a natural number.
Then, x² × 3 – 4x = x + 50
3x² – 4x – x = 50
3x² – 5x = 50
3x² – 5x – 50 = 0
3x² + 10x – 15x – 50 = 0
x (3x + 10) – 5 (3x + 10) = 0
(x – 5) (3x + 10) = 0
x – 5 = 0 , 3x + 10 = 0
x = 5 or 3x = – 10
x = 5 or x = – 10/3 (not natural number.
Ans. x = 5 is a natural number.
Q.8 On subtracting the square of a natural number from its cube, the result is 48. will be the number?
Solution : According to the question,
Let x be a natural number.
x³ – x² = 48
x² (x – 1) = 48
x² (x – 1) = 16 × 3
4² × (4 – 1)
x = 4
Q.9 Subtracting the square of a natural number from its cube gives 100. is that number?
Solution : According to the question,
Let x be a natural number.
x³ – x² = 100
x² (x – 1) = 100
x² (x – 1) = 25 × 4
5² × (5 – 1)
x = 5
Q.10 What is the sum of cubes of all natural number from 1 to 100?
A. 12,867,900
B. 18,234,400
C. 27,856,908
D. 25,502,500
Solution : According to the question,
[n (n + 1) / 2]²
n = 100
[100 (100 + 1) / 2]²
[(100 × 101) / 2]²
(10,100 / 2)²
(5,050)²
Ans. 25,502,500
FAQ
1. What are the first twenty natural number?

2. Is every whole number a natural number?
Answer : All whole number except zero are natural number. Because the value of a whole number ranges from zero to infinity. The same natural number range from one to infinity.
3. How many natural number are there between 25 and 36?
Answer : There are 10 natural number between 25 and 36. (26, 27, 28, 29, 30, 31, 32, 33, 34, 35)
4. Find the average of natural number from 1 to 100?
Answer : Average of numbers = Sum of total numbers / Total numbers
Average of numbers = (1+100)/2
= 101/2
= 50.5
Ans. 50.5
5. Find the sum of natural number from 1 to 100?
Answer : Sum of natural number = Average of number × number
Sum of natural number = 50.5×100
= 5050
Ans. 5050
6. Average of even numbers from 1 to 100?
Answer : Average of the numbers = Sum of the total numbers × Total numbers
Average of numbers = (2+100)/2
= 102/2
= 51
Ans. 51
7. Sum of even natural numbers from 1 to 100?
Answer : Sum of natural number = Average × Total number
Sum of natural even number = 51 × 51
= 2550
Ans. 2550
8. Sum and average of odd numbers from 1 to 100?
Answer : Average of odd numbers = Sum of total numbers / Total numbers
Average of odd numbers = sum of numbers × total numbers
Average of odd numbers = (1 + 99)/2
= 100/2
= 50
Sum of odd numbers = 50×50
= 2500
Ans. 2500
9. What is the smallest natural number?
Answer : The smallest natural number is 1.
10. Which is the largest natural number?
Answer : There is no largest natural number. Because the natural number range from 1 to infinity.
Must Read :
Hope you have liked the information about natural numbers.
Comment for any question related to natural numbers.