Definition, Formula and Examples of LCM and HCF

On this page, you will understand the information about LCM and HCF important chapters of Mathematics subject.

In the previous post, we have shared all the information about the Number System Definition, Types & Example, if you have not read it yet, then definitely read it.

Let us understand LCM and HCF in detail below.

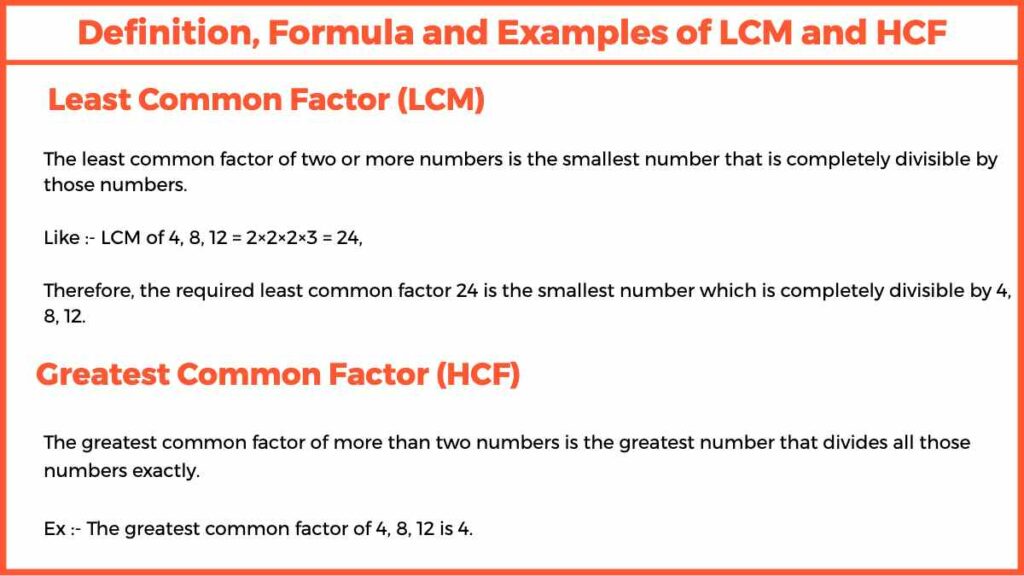
Least Common Factor (LCM)

The least common factor of two or more numbers is the smallest number that is completely divisible by those numbers.

Like :- LCM of 4, 8, 12 = 2×2×2×3 = 24,

Therefore, the required least common factor 24 is the smallest number which is completely divisible by 4, 8, 12.

Greatest Common Factor (HCF)

The greatest common factor of more than two numbers is the greatest number that divides all those numbers exactly.

Ex :- The greatest common factor of 4, 8, 12 is 4.

Formulas for LCM and HCF

• Least Common Factor of Fractions (L.C.M.) = Least Common Factor of the numerator / Greatest Common Factor of the denominator

• Greatest Common Factor of Fractions (H.C.F.) = Greatest Common Factor of the Numerator / Least Common Common Factor of the Denominator

• L.C.M × H.C.F = First Number × Second Number

• LCM = least common multiple of numerator / greatest common multiple of denominator

• HCF = Greatest Common Factor of the numerator / Smallest Common Factor of the denominator

Examples of LCM and HCF

Q.1 Find the least common and greatest common factor of 24, 32, 40 by factorization method?
A. 120, 2
B. 280, 4
C. 360,6
D. 480, 8

Ans. 480, 8

Q.2 Find the greatest common factor of 18, 42, 102?
A. 12
B. 2
C. 6
D. 8

Solution:- According to the question,
18 = 2 × 3 × 3
42 = 2 × 3 × 7
102 = 2 × 3 × 17
The common prime factors are 2 and 3.
Required greatest common factor = 2 × 3
= 6

Ans. HCF = 6

Q.3 Find the HCF of 60, 45, 180, 24?
A. 2
B. 3
C. 5
D. 8

Solution:- According to the question,
60 = 2 × 2 × 3 × 5
45 = 3 × 3 × 5
180 = 2 × 2 × 3 × 3 × 5
24 = 2 × 2 × 2 × 3
The common prime factors are 3.
Required greatest common factor = 3

Ans. 3

Q.4 Find the HCF of 923, 949?
A. 12
B. 13
C. 16
D. 18

Solution:- According to the question,
923 = 13 × 71
949 = 13 × 73
The common prime factors are 13.
Required greatest common factor = 13

Ans. 13

Q.5 Find the greatest common factor of 216, 288 and 720?
A. 120
B. 280
C. 360
D. 480

Solution:- According to the question,
216 = 2 × 2 × 2 × 3 × 3 × 3
288 = 2 × 2 × 2 × 2 × 2 × 3 × 3
720 = 2 × 2 × 2 × 2 × 3 × 3 × 5
The common prime factors are 2 and 3.
Required greatest common factor = (2 × 2 × 2 × 3 × 3)
= 72

Ans. 72

Q.6 Find the greatest common factor of 9/10, 12/25, 18/35, 21/40?
A. 12/700
B. 28/3200
C. 6/1400
D. 48/4800

Solution: HCF = 9, 12, 18, 21
= 2 × 3
= 6
LCM = 2 × 2 × 2 × 5 × 5 × 7
LCM = 1400
HCF = HCF of the numerator / LHC of the denominator
HCF = 6/1400

Ans. 6/1400

Q.7 Find the least common factor of 3/4, 6/7, 8/9?
A. 24
B. 3
C. 3/56
D. 8

Solution:- According to the question,
LCM of 3/4, 6/7, 8/9
= (LCM of 3, 6, and 8)/(LCM of 4, 6, and 7)
= 24/1
24

Ans. 24

Q.8 What is the least common factor of 5/7, 7/8 and 8/9?
A. 120
B. 280
C. 360
D. 480

Solution:- According to the question,
LCM of 5/7, 7/8 and 8/9
Abhishta L. S. = (LCM of 5, 7, 8)/(LCM of 7,8,9)
280

Ans. 280

Q.9 The smallest fraction which is completely divisible by 6/7, 5/14, 10/21 is?
A. 30/98
B. 60/90
C. 30/7
D. 60/147

Solution:- According to the question,
Abhishta L. S. = (L.C.S. of 6,5,10)/(L.C.S. of 7,14,21)
30/7

Ans. 30/7

Q.10 What is the least common factor of 0.9, 0.18, 3.6, 7.2, 0.144?
A. 1.44
B. 7.2
C. 12.96
D. 18.32

Solution:- According to the question,
0.9, 0.18, 3.6, 7.2, 0.144
There are at most three digits after the decimal.
Therefore multiplying all the numbers by 1000 gives the number
= 900, 180, 3600, 7200 and 144
L of these numbers S. = 7200
Abhishta L. S. = 7200/100
LCM = 7.2

Ans. 7.2

Q.11 L.C. of x² + xy + y² and x³ – y³ Will M.
A. x – y
B. x² – y²
C. x³ – y³
D. x² + xy + y²

Solution:- According to the question,
x² + xy + y² =
x³ – y³ = (x – y)(x² + xy + y²)
Required L.C.M. = (x – y) (x² + xy + y² )

Ans. x³ – y³

Q.12 The greatest common factor of x² – 9 and x² – 6x + 9 is?
A. x – 3
B. x + 3
C. x + 2
D. x – 2

Solution:- According to the question,
x² – 9 = (x – 3)(x – 3)
x² – 6x + 9 = x² – 3x – 3x + 9
x(x – 3) – 3(x – 3)
(x – 3)(x – 3)
Required greatest common factor = (x – 3)

Ans. (x – 3)

Q.13 Find the LCM and GCM of 7/9, 14/15, 7/10?
A. 120, 8
B. 28, 12
C. 90, 7
D. 18, 3

Solution:- According to the question,
Abhishta L. S. = (LCS of 7,14,7)/(LCS of 9,15,10)
90/7

Ans. LCM = 90, HCF = 7

Q.14 What is the simplest form of 368/437?
A. 12/17
B. 16/19
C. 9/14
D. 18/23

Solution:- According to the question,
HCF = 23
368 / 23 = 16
437 / 23 = 19
The simplest form would be 16/19.

Ans. 16/19

Q.15 The traffic signals at three different intersections change after 48 seconds, 72 seconds, and 108 seconds respectively. If they are changed together now, then after how much time will they change?
A. 165
B. 285
C. 432
D. 350

Solution:- According to the question,
L.C.M. of 48, 72, 108 = 432

Ans. 432

Q.16 The length of 3 types of wires are 4672, 3869, 2993, meters respectively, what is the maximum length of another type of wire so that the length of all the three types of wires can be measured exactly.
A. 16
B. 28
C. 43
D. 73

Solution:- According to the question,
HCF of 4672, 3869, 2993 = 73

Ans. 73

Q.17 The LSA of two numbers is 495 and their greatest common factor is 5. If the sum of those numbers is 100, then what will be their difference?
A. 5
B. 10
C. 15
D. 20

Solution:- According to the question,
Let first number = a
second number = b
Formula – First number × second number = L.S × M.S
a × b = 495 × 5
ab = 2475
according to the question,
a + b = 100
Formula – (a – b) ² = ( a + b ) ² – 4ab
= (100)² – 4 × 2475
= 10000 – 9900
(a – b) ² = 100
(a – b ) = 10

Ans. 10

Q.18 Find the least number which when divided by 5, 6, 7, 8 leaves a remainder of 3 but when divided by 9 leaves no remainder?
A. 1560
B. 1683
C. 1820
D. 1960

Solution :- According to the question,
LCM of 5, 6, 7, 8 = 840
Therefore, the required number = (840 × n + 3).
where n is any natural number.
The least value of n such that (840 × n + 3) is divisible by 9 is
That minimum value will be n = 2.
Required number = 840 × 2 + 3
Required number = 1683

Ans. 1683

Q.19 Find the least number which when divided by 10, 20, 30, 40 and 50 leaves a remainder 7 in each case?
A.607
B.709
C.504
D.810

Solution:- According to the question,
Formula :- Tell the least number which when divided by x, y, z and p leaves the same remainder (k) in each case, then the number = L.S. (x, y, z, p) + k)
Required number = L.S. (10, 20, 30, 40, 50) + 7
= 600 + 7
= 607

Ans. 607

Q.20 Find the smallest four digit number which is exactly divisible by 2, 3, 4, 5, 6 and 7?
A.1270
B.1260
C.1570
D.1470

Solution:- According to the question,
First of all we find the LCM of 2, 3, 4, 5, 6 and 7. will remove
L.S. (2, 3, 4, 5, 6 and 7) = 420
Since we need a 4 digit number, the required number must be a multiple of 420.
Required number = 420 x 3
= 1260

Ans. 1260

FAQ

Read More :
Simplification	Profit and Loss
Simple Interest	Compound Interest

Hope you have liked the information about LCM and HCF. If you liked this information then definitely share it with your friends.