On this page you will read the information of a+b Whole Cube.
a+b Whole Cube
The (a + b)3 formula is used to find the cube of a binomial. This formula is also used to factorize some special types of trinomials. This formula is :
- one of the algebraic identities.
- the formula for the cube of the sum of two terms.
Let us understand (a + b)3 formula in detail in the following section.

What Is the (a + b)3 Formula?
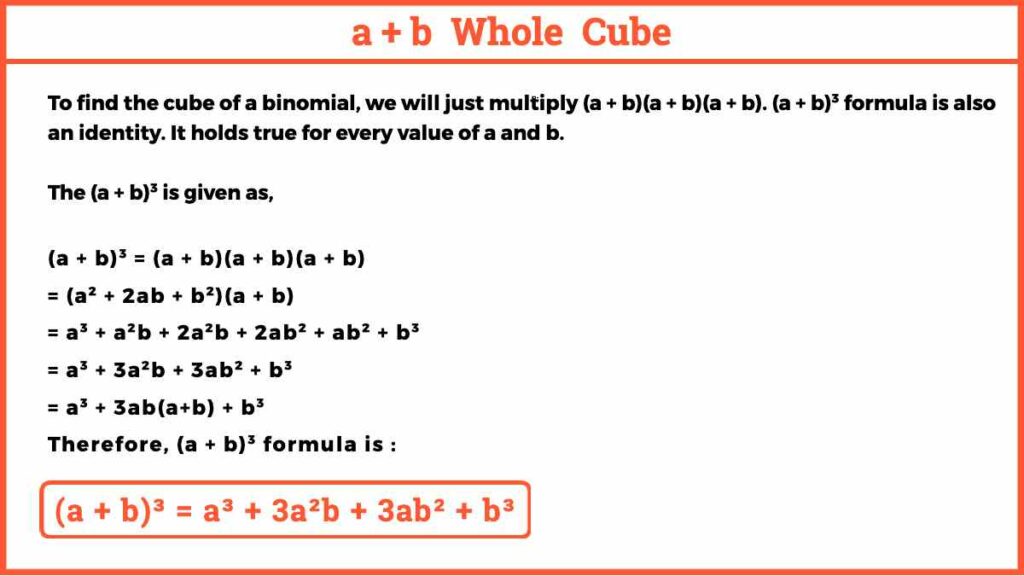
To find the cube of a binomial, we will just multiply (a + b)(a + b)(a + b). (a + b)3 formula is also an identity. It holds true for every value of a and b.The (a + b)3 is given as,
(a + b)3 = (a + b)(a + b)(a + b)
= (a2 + 2ab + b2)(a + b)
= a3 + a2b + 2a2b + 2ab2 + ab2 + b3
= a3 + 3a2b + 3ab2 + b3
= a3 + 3ab(a+b) + b3
Therefore, (a + b)3 formula is :
(a + b)3 = a3 + 3a2b + 3ab2 + b3
Examples on (a + b)3 Formula
Example 1. Solve the following expression using suitable algebraic identity: (2x + 3y)3
Solution :
To find : (2x + 3y)3
Using (a + b)3 Formula,
(a + b)3 = a3 + 3a2b + 3ab2 + b3
= (2x)3 + 3 × (2x)2 × 3y + 3 × (2x) × (3y)2 + (3y)3
= 8x3 + 36x2y + 54xy2 + 27y3
Answer : (2x + 3y)3 = 8x3 + 36x2y + 54xy2 + 27y3
Example 2. Find the value of x3 + 8y3 if x + 2y = 6 and xy = 2.
Solution :
To find: x3 + 8y3
Given: x + 2y = 6
xy = 2
Using (a + b)3 formula,
(a + b)3 = a3 + 3a2b + 3ab2 + b3
Here, a = x; b = 2y
Therefore,
(x + 2y)3 = x3 + 3 × x2 × (2y) + 3 × x × (2y)2 + (2y)3
(x + 2y)3 = x3 + 6x2y + 12xy2 + 8y3
63 = x3 + 6xy(x + 2y) + 8y3
216 = x3 + 6 × 2 × 6 + 8y3
x3 + 8y3 = 144
Answer: x3 + 8y3 = 144
Example 3. Solve the following expression using (a + b)3 formula : (5x + 2y)3
Solution :
To find: (5x + 2y)3
Using (a + b)3 Formula,
(a + b)3 = a3 + 3a2b + 3ab2 + b3
= (5x)3 + 3 × (5x)2 × 2y + 3 × (5x) × (2y)2 + (2y)3
= 125x3 + 150x2y + 60xy2 + 8y3
Answer: (5x + 2y)3 = 125x3 + 150x2y + 60xy2 + 8y3
FAQ
Ans. (a + b) whole cube formula says: (a + b)3 = a3 + 3a2b + 3ab2 + b3. To find the cube of a binomial, we will just multiply (a + b)(a + b)(a + b). (a + b)3 formula is also an identity. It holds true for every value of a and b.
Ans. A polynomial equation, commonly called an algebraic expression, is a mathematical formula of the type : P=0. Formulas for a3+b3 are : a3 + b3 = (a + b) (a² – ab + b²)
Ans. Length = Breadth = Height = a. Thus, the measure of each edge of the cube = a. Therefore, the volume of cube formula is a × a × a = a3.
Ans. α – β = √(α + β)² – 4αβ
Ans. (a + b)3 formula is also an identity. It holds true for every value of a and b. Therefore, (a + b)3
Formula is : (a + b)3 = a3 + 3a2b + 3ab2 + b3.
Ans. The value of cube 1 to 30 is the list of numbers obtained by multiplying the same integer three times.
For Example, 13 = 1×1×1 = 1, 23 = 2×2×2 = 8, 33 = 3×3×3 = 27, 43 = 4×4×4 = 64, and so on.
Must Read :
Hope You Have Liked the Information About a+b Whole Cube.